Like many mathematical terms, the word perimeter has its origins in the work of early Greek mathematicians. It is derived from the Greek words ‘peri’, which means ‘around’ and ‘metron’, which means ‘a measurement’. Perimeter is literally a measurement around.

In everyday usage, you may have come across phrases such as perimeter fence, estate perimeter, or perimeter security. These mean that the fence, or the security provision, are around the edges, the outer limits or extremities of a measured area of land or property.

Understanding how to calculate perimeter is a useful mathematical skill for both study and real life, whether performing geometric calculations, marking out a playing field or replacing a fence.

---

Measuring Perimeter of Regular Polygons

The perimeter of a two-dimensional shape is the total length of all the sides added together.

For example, the perimeter of a square, with a side length of 6m, is simply four lots of 6m, i.e. 4 × 6m = 24m. The square has four sides of equal length, which are added together.

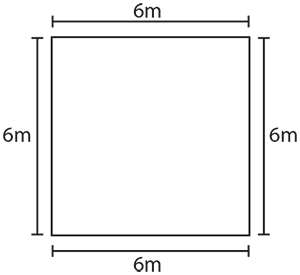
A square of any side length s therefore has a perimeter equal to 4 × s, or simply 4s .

You can use the same principle to work out the perimeter of any regular polygon that has any number of sides of equal length:

If your polygon has n number of sides, all of length s, then its perimeter is always equal to n × s, or simply ns.

So for example, if you have a heptagon (7 sides) with side length 15cm, then the length of the perimeter is 7 × 15 = 105cm.

For more on regular, irregular and other polygons (straight-sided shapes), including a helpful chart with illustrations, see our page on properties of polygons.

Measuring Perimeter of Irregular Polygons
A regular polygon has all sides and internal angles equal, irregular polygons do not.

A rectangle that is not an exact square, for example, has two pairs of sides of equal length, but all four sides are not the same length.

Example

Find the perimeter of a rectangular football pitch, measuring 105 × 68 m.

The lengths of the opposite sides are equal to each other, so you need to add together two lots of 105m and two lots of 68m.

2 × 105 = 210m
2 × 68 = 136m
210 + 136 = 346m

The perimeter of the pitch is 346m.

---

Irregular polygons can be formed by any combination of straight lines that join up to enclose an area. No matter how complicated the shape, the perimeter will always be the sum of the lengths of the sides.

The shape below could be a garden plot, or anything else you can think of. In geometrical terms, it is an eight-sided enclosed 2D shape, which has no sides of the same length, and no internal angles of the same size.

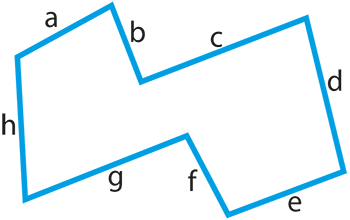
It is an irregular octagon (8 sides) and its perimeter is equal to a + b + c + d + e + f + g + h.

---

Example

Calculate the perimeter of the template below, dimensions are in inches.

Starting at the bottom left corner and working your way around the shape in a clockwise direction, add up the lengths of the sides:

5 + 2 + 3 + 4 + 2 + 3 + 4 + 9 = 32 inches.

The perimeter of the shape is 32 inches.

---

---

Circumference

Circumference is a specific type of perimeter that applies exclusively to circular shapes.

Measuring the circumference of a circle

The mathematical expression for calculating the circumference of a circle is:

2 × π × radius  or simply 2πr

The diameter of a circle is equal to twice the radius, so the expression for circumference can also be written πD.

Measuring the circumference of an ellipse

Not all curved shapes are perfectly circular and sometimes it might be necessary to find the perimeter of an ellipse (a squashed or elongated circle).

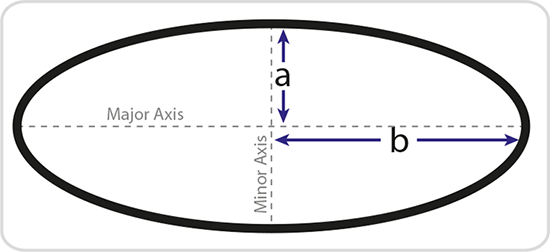
The perimeter:

$$p \approx 2\pi\sqrt{\frac{a^2+b^2}{2}}$$

You can see that this expression is similar to the expression for the circumference of a circle, but radius r is replaced by \(\sqrt{\frac{a^2+b^2}{2}}\), where a and b are half the length of the minor axis and major axis respectively. (For more on ellipses, see our page on circles and curved shapes).

This equation only provides an approximation (≈). The more elongated the ellipse becomes, the more inaccurate the answer. Mathematicians have come up with several complex formulae for solving this problem. None of them have achieved 100% accuracy in a mathematical sense, but it is unlikely that you would need such a high level of precision unless you work in engineering or design.

---

---

Conclusion

Perimeter is the mathematical term used to define the total length of the edges of a multisided two-dimensional enclosed shape (polygon). In the case of circular shapes, it is called a circumference.

Many professions require these mathematical skills, often used in conjunction with much more complex geometry and trigonometry. However, a basic understanding of the principles will allow you perform jobs around the house and garden with more mathematical confidence. You will now be able to work out how many bricks are required to go around the edge of a circular pond!

---