Curved Shapes

Circles, Ellipses, Parabolas and Hyperbolas

Our page on Polygons covers shapes made with straight lines, also known as ‘plane shapes’. This page explains more about shapes with curves, especially two-dimensional ones.

Two-dimensional curved shapes include circles, ellipses, parabolas, and hyperbolas, as well as arcs, sectors and segments. Three-dimensional curved shapes, including spheres, cylinders and cones, are covered on our page on Three-Dimensional Shapes.

Two-Dimensional Curved Shapes

Properties of a circle. Circumference, Diameter and Radius.

Circles

Probably the most common two-dimensional curved shape is a circle.

In order to work with circles (and other curved shapes) in geometry it is important to understand the key properties of a circle:

A line straight across the centre of a circle is the diameter.
Half of the diameter is the radius.
The line around the edge of a circle is the circumference.

Any point on the circumference of a circle is exactly the same distance away from the centre of the circle as any other point on the circumference.

Introducing π (pi)

π or pi is a Greek letter. In mathematics, it is used to represent a particular constant, which is also an irrational or infinite number (see our page on Special Numbers for more).

π has a value of 3.142 (although as it is infinite, this is an approximation of its exact value).

π is important because it is used to calculate the circumference and the area of a circle.

The circumference of a circle is equal to π x diameter, or 2 × π × radius (abbreviated to 2πr).

The area of a circle is equal to π × radius². This formula is usually abbreviated to πr²

For more about area, see our page Calculating Area.

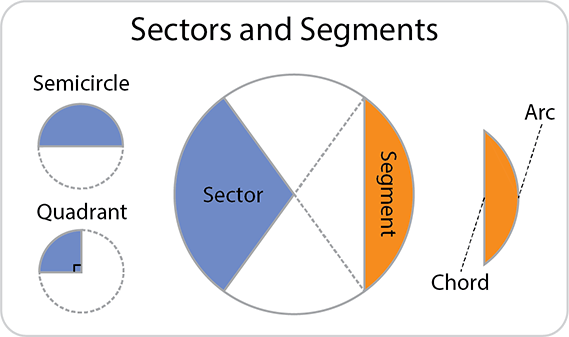
Sectors and Segments

Sectors and segments are 'slices' of a circle.

Sectors are shaped like a slice of pizza, with a curved edge and each straight side the same length as the radius of the circle, or pizza, from which it was cut. Pie charts are made up of a number of sectors relating in size to the data they show.

A sector can be any size, however a sector that is half a circle (180°) is called a semicircle, while a quarter circle sector (90°) is called a quadrant.

A segment is the curved part of a sector, the part that is left if you remove the triangle from a sector. Segments are made up of two lines. The arc (a section of the circumference of the circle - see below) and a chord - the straight line joining the two ends of the arc.

Circle sectors including semicircles (half-circles) and quadrants (quarter circles). Segments of a circle, chord and arc.

A sector is a fraction of a circle and therefore its area is a fraction of the area of the whole circle. To calculate the area of a sector you need to know its central angle, θ and the radius.

The area of a sector can then be calculated by using the following formula:

πr² × (θ ÷ 360)

Arcs

Length of an arc of a circle. 2πr × (θ ÷ 360)

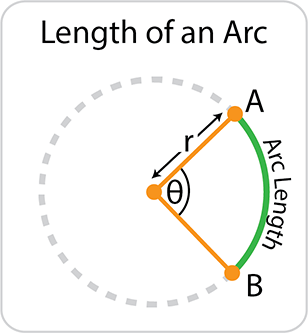
A section of the circumference of a circle is called an arc.

To calculate the length of an arc between points A and B, you need to know the angle at the centre between points A and B. θ (theta) is the symbol used to represent this angle subtended by A and B. In our example, we are using degrees for θ, but it is also possible to use radians.

You also need to know the radius (r) of the arc.

As there are 360° in the whole circle, the length of the arc is equal to the central angle (θ) divided by 360, then multiplied by the circumference of the whole circle (2πr).

2πr × (θ ÷ 360)

Example:

r = 10cm, θ = 88°, π = 3.14

Arc Length = 2 x 3.14 x 10 x (88 ÷ 360) = 62.8 × 0.24 = 15.07cm.

Degrees or Radians?

The most commonly used unit of measurement for angles is degrees, but you may also come across calculations where the angle is measured in radians. This is the standard SI unit for the measurement angles, and for more information about radians, see our Introduction to Angles page. For more information about the SI measurement system, see our page on Systems of Measurement.

2π radians is equal to 360°, so the formula for arc length when θ is in radians is simply rθ.

Ellipses

An ellipse is a curve on a plane (or flat surface) surrounding two focal points. A straight line drawn from one focal point to any point on the curve and then to the other focal point has the same length for every point on the curve.

Ellipses are very important in astronomy and physics, since every planet has an elliptical orbit with the sun as one of the focal points.

A circle is a specific form of ellipse, where the two focal points are in the same place (at the circle's centre). Ellipses may also be described as ‘oval’, but the word ‘oval’ is much less precise in maths, and simply means ‘broadly egg-shaped’.

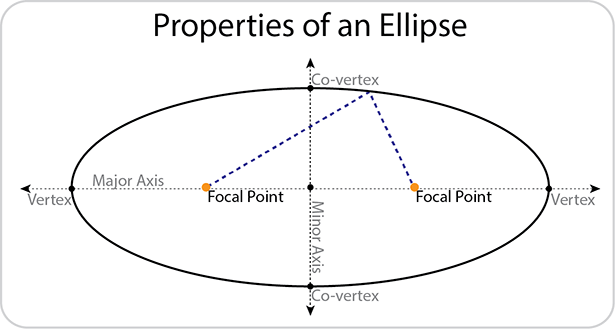
Properties of an ellipse:

An ellipse has two main axes, and is symmetrical around them.

The longer axis is called the major axis; the shorter axis is the minor axis.

The four points where the axes cross the circumference are called the vertices (singular vertex). The two points where the minor axis crosses the circumference are called the co-vertices.

The two focal points (or foci, sometimes called locus or loci) are both on the major axis, and equal distances away from the centre.

The distance from one focal point to any point on the circumference, and back to the other focal point (the blue dotted line in our diagram) is the same as the length between the vertices on the major axis.

The extent to which an ellipse is elongated is defined by its eccentricity. The formula for calculating the eccentricity is:

Eccentricity =	distance from centre to focal point
	distance from centre to vertex on the major axis

The eccentricity of a circle is zero, because the focal points are in exactly the same place (the centre) (we also say that they are coincident). The distance from the centre to the focal point is therefore zero. The eccentricity increases as the ellipse becomes longer, but is always less than 1. When the distance from the centre to the focal point is the same as the distance from the centre to the vertex, then the ellipse has become a straight line and its eccentricity is equal to 1.

The area of an ellipse is calculated as π (½ x minor axis)(½ x major axis).

Parabolas, Hyperbolas and the Relationship Between Curved Shapes

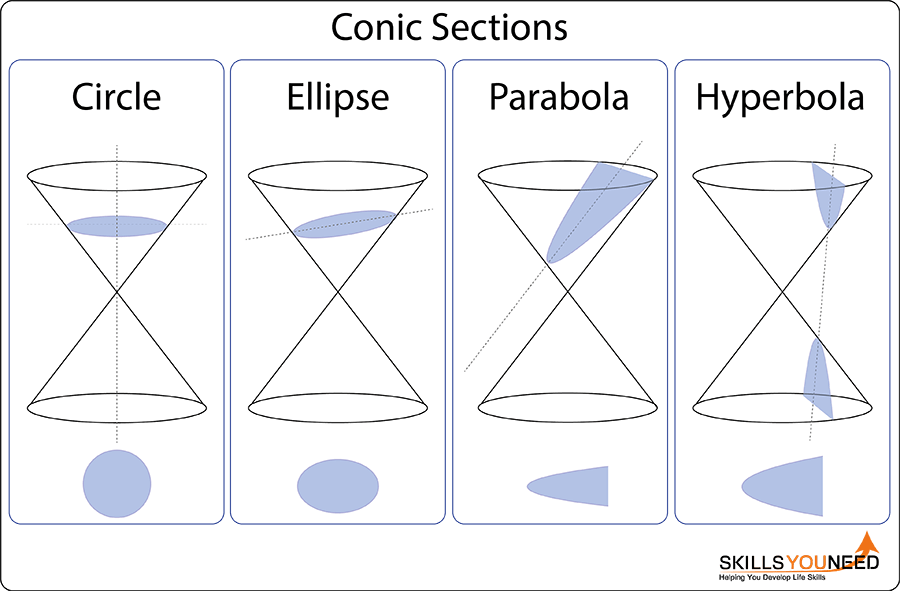
Parabolas and Hyperbolas are more forms of curved shapes, but they are more complicated to define than circles and ellipses. They are closely related to each other and to circles and ellipses, because they are all conic sections, i.e. shapes that are formed by slicing through a cone with a flat plane.

The characteristics of conic sections have been studied for millennia and were a subject of interest for ancient Greek mathematicians such as Euclid and Archimedes. The diagram below shows a double cone, rather like a sand-timer.

If the plane cuts the cone at an angle parallel to the base of the cone (i.e. perpendicular to its vertical axis), then a circle is formed (top left).
If the plane cuts the cone parallel to the side of the cone, then a parabola is formed (centre).
If the plane cuts the cone at an angle between these two, such that it maintains contact with the sides of the cone in all locations, then an ellipse is formed (bottom left).
If the plane cuts through both cones at a more vertical angle, then the section is a hyperbola.

Parabolas and hyperbolas are both symmetrical curves with a single axis of symmetry and a vertex (lowest point of the curve’s u-shape).

All parabolas have the same characteristic shape, no matter how big they are. As you zoom out further and further from the vertex towards infinity, the parabola changes from a bowl shape to a hairpin shape, with its arms becoming closer and closer to parallel.

Unlike parabolas, hyperbolas can be different shapes, because the angle of the cut can vary widely. Both parabolas and hyperbolas are infinite, but a hyperbola’s arms never become parallel.

Conic Sections. How a cone can be cut to produce, a circle, an ellipse, a parabola or a hyperbola.

Real-world applications of conical sections

There are many real-world applications of conical sections.

They are used in lenses for telescopes and the reflectors in headlights or spotlights to create a beam of light.
The complex maths associated with these shapes is vital in the calculation of the orbits of satellites.
In engineering, the cables on the Golden Gate Bridge are in the shape of perfect parabolas, and the aerofoils in aircraft are based on ellipses.
In sports, the arc followed by a football, baseball or cricket ball is also a parabola, so understanding conical sections is vital for analysing player performance—increasingly important with the money invested in professional sports.
The organic form of these shapes also lends them to uses in the arts and architecture. Examples include the Cybertecture Egg in Mumbai, the Gateway Arch in Missouri and numerous sculptural artists’ work, such as Richard Serra’s Torqued Ellipses at the Guggenheim museum in New York City.

Skills You Need?

Circles are part of basic geometry, and you really need to know how to calculate basic properties of them.

It is, however, probably unlikely that you would need to do more than be aware of the existence of the other shapes unless you wished to get seriously into engineering, physics, or astronomy.

That said, you may find that you appreciate knowing that the concave curves of a power station cooling tower, or the light from a downward-pointing halogen lamp, are in the shape of a hyperbola.

Understanding Geometry - The Skills You Need Guide to Numeracy