Averages: Mean, Median and Mode

Mean, Median and Mode Calculator

Use this calculator to work out the mean, median and mode of a set of numbers.

Mean

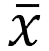
The mathematical symbol or notation for mean is ‘x-bar’. This symbol appears on scientific calculators and in mathematical and statistical notations.

The ‘mean’ or ‘arithmetic mean’ is the most commonly used form of average. To calculate the mean, you need a set of related numbers (or data set). At least two numbers are needed in order to calculate the mean.

The numbers need to be linked or related to each other in some way to have any meaningful result – for instance, temperature readings, the price of coffee, the number of days in a month, the number of heartbeats per minute, students’ test grades etc.

To find the (mean) average price of a loaf of bread in the supermarket, for example, first record the price of each type of loaf:

White: £1
Wholemeal: £1.20
Baguette: £1.10

Next, add (+) the prices together £1 + £1.20 + £1.10 = £3.30

Then divide (÷) your answer by the number of loaves (3).

£3.30 ÷ 3 = £1.10.

The average price of a loaf of bread in our example is £1.10.

The same method applies with larger sets of data:

To calculate the average number of days in a month we would first establish how many days there are in each month (assuming that it was not a leap year):

Month	Days
January	31
February	28
March	31
April	30
May	31
June	30
July	31
August	31
September	30
October	31
November	30
December	31

Next we add all the numbers together: 31 + 28 + 31 + 30 + 31 + 30 + 31 + 31 + 30 + 31 + 30 + 31 = 365

Finally we divide the answer with the number of values in our data set in this case there are 12 (one for each month counted).

So the mean average is 365 ÷ 12 = 30.42.

The average number of days in a month, therefore, is 30.42.

The same calculation can be used to work out the average of any set of numbers, for example the average salary in an organisation:

Let's assume the organisation has 100 employees on one of 5 grades:

Grade	Annual Salary	Number of Employees
1	£20,000	21
2	£25,000	25
3	£30,000	40
4	£50,000	9
5	£80,000	5

In this example we can avoid adding each individual employee’s salary as we know how many are in each category. So instead of writing out £20,000 twenty-one times we can multiply to get our answers:

Grade	Annual Salary	Number of Employees	Salary x Employees
1	£20,000	21	£420,000
2	£25,000	25	£625,000
3	£30,000	40	£1,200,000
4	£50,000	9	£450,000
5	£80,000	5	£400,000

Next add the values in the Salary x Employees column to find a total: £3,095,000 and finally divide this number by the number of employees (100) to find the average salary:

£3,095,000 ÷ 100 = £30,950.

Quick Tip:

The salaries, in the example above, are all multiples of £1,000 – they all end in ,000.

You can ignore the ,000's when calculating as long as you remember to add them back on at the end.

In the first row of the table above we know that twenty-one people get paid a salary of £20,000, instead of working with £20,000 work with 20:

21 x 20 = 420 then replace the ,000 to get 420,000.

Sometimes we may know the total of our numbers but not the individual numbers that make up the total.

In this example, assume that £122.50 is made by selling lemonade in a week.

We don’t know how much money was made each day, just the total at the end of the week.

What we can work out is the daily average: £122.50 ÷ 7 (Total money divided by 7 days).

122.5 ÷ 7 = 17.50.

So we can say that on average we made £17.50 a day.

We can also use averages to give us a clue of likely future events – if we know that we made £17.50 a day on average selling lemonade in a week then we can assume that in a month we would make:

£17.50 × Number of days in that month

17.50 × 31 = £542.50

We could record average sales figures each month to help us predict sales for future months and years and also to compare our performance. We could use terms like ‘above average’ – to refer to a time period when sales were more than the average amount and likewise ‘below average’ when sales were less than the average amount.

Using speed and time as data to find the mean:

If you travel 85 miles in 1 hour and 20 minutes, what was your average speed?

The first thing to do with this problem is to convert the time into minutes – time does not work on the decimal system as there are 60 minutes in an hour and not 100. Therefore we need to standardise our units before we can start:

1 hour 20 minutes = 60 minutes + 20 minutes = 80 minutes.

Next divide the distance travelled by the time taken: 85 miles ÷ 80 minutes.

85 ÷ 80 = 1.0625.

Our average speed therefore was 1.0625 miles per minute.

Convert this figure back to hours by multiplying by 60 (the number of minutes in an hour).

1.0625 × 60 = 63.75mph (miles per hour).

For Spreadsheet users:

Use the <average> function to calculate the mean average in a spreadsheet. The following example formula, assumes your data is in cells A1 to A10:

=average(A1:A10)

Median

The Median is the middle number in a list of sorted numbers.

To calculate the Median of: 6, 13, 67, 45, 2

First, arrange the numbers in order (this is also known as ranking)

2, 6, 13, 45, 67

then - find the middle number

Median = 13, the middle number in the ranked list.

When there are an even number of numbers there is no single middle number but a pair of middle numbers.

In such cases the median is the mean of the two middle numbers:

For example:

6, 13, 67, 45, 2, 7.

Arranged in order (ranked) = 2, 6, 7, 13, 45, 67

The middle numbers are 7 and 13.

The median refers to a single number so we calculate the mean of the two middle numbers:

7 + 13 = 20
20 ÷ 2 = 10

Therefore the median of 6, 13, 67, 45, 2, 7 is 10.

Mode

The Mode is the most frequently-occurring value in a set of values. The mode is interesting as it can be used for any type of data, not just numbers.

In this example, assume that you have bought a pack of 100 balloons, the pack is made up of 5 different colours, you count each colour and find that you have:

18 Red
12 Blue
24 Orange
25 Purple
21 Green

The mode of our sample of balloons is purple as there are more purple balloons (25) than any other colour balloon.

To find the mode of the number of days in each month:

Month	Days
January	31
February	28
March	31
April	30
May	31
June	30
July	31
August	31
September	30
October	31
November	30
December	31

7 months have a 31 days, 4 months have a total of 30 days and only 1 month has a total of 28 days (29 in a leap year).

The mode is therefore, 31.

Some data sets may have more than one Mode:

1,3,3,4,4,5 – for example, has two most frequently occurring numbers (3 & 4) this is known as a bimodal set. Data sets with more than two modes are referred to as multi-modal data sets.

If a data set contains only unique numbers then calculating the mode is more problematic.

It is usually perfectly acceptable to say there is no mode, but if a mode has to be found then the usual way is to create number ranges and then count the one with the most points in it. For example from a set of data showing the speed of passing cars we see that out of 10 cars the recorded speeds are:

40, 34, 42, 38, 41, 50, 48, 49, 33, 47

These numbers are all unique (each only occurs once), there is no mode. In order to find a mode we build categories on an even scale:

30--32 | 33--35 | 36--38 | 39--41 | 42--44 | 45--47 | 48--50

Then work out how many of the values fall into each category, how many times a number between 30 and 32 occurs, etc.

30--32 = 0
33--35 = 2
36--38 = 1
39--41 = 2
42--44 = 1
45--47 = 1
48--50 = 3

The category with the most values is 48--50 with 3 values.

We can take the mid value of the category to estimate the mode at 49.

This method of calculating the mode is not ideal because the mode may change depending on the categories you define.

Data Handling and Algebra - The Skills You Need Guide to Numeracy